Regularity In Math Examples - $m$ is regular if, for any $a\in\mathcal{a}$, the measure of $a$ equals the infimum of measures. Every elementary function is continuous. This includes all rational functions, which are built up from combinations of the function x with. A regularity condition is essentially just a requirement that whatever structure you are studying isn't too poorly behaved. Very recently, a new theory of “regularity structures” was introduced [hai14], unifying various flavours of the theory of (controlled) rough paths (including. The standard definition of regularity goes like this: While many problems or tasks do require students to use a combination of. The regularity a solution can inherit depends on the properties of the problem, i.e., the smoothness of a domain boundary, the. Look for and express regularity in repeated reasoning.
This includes all rational functions, which are built up from combinations of the function x with. Very recently, a new theory of “regularity structures” was introduced [hai14], unifying various flavours of the theory of (controlled) rough paths (including. While many problems or tasks do require students to use a combination of. $m$ is regular if, for any $a\in\mathcal{a}$, the measure of $a$ equals the infimum of measures. Look for and express regularity in repeated reasoning. A regularity condition is essentially just a requirement that whatever structure you are studying isn't too poorly behaved. The regularity a solution can inherit depends on the properties of the problem, i.e., the smoothness of a domain boundary, the. Every elementary function is continuous. The standard definition of regularity goes like this:
The regularity a solution can inherit depends on the properties of the problem, i.e., the smoothness of a domain boundary, the. $m$ is regular if, for any $a\in\mathcal{a}$, the measure of $a$ equals the infimum of measures. A regularity condition is essentially just a requirement that whatever structure you are studying isn't too poorly behaved. This includes all rational functions, which are built up from combinations of the function x with. The standard definition of regularity goes like this: Very recently, a new theory of “regularity structures” was introduced [hai14], unifying various flavours of the theory of (controlled) rough paths (including. While many problems or tasks do require students to use a combination of. Every elementary function is continuous. Look for and express regularity in repeated reasoning.
(PDF) A regularity theory for an initial value problem with a time
The standard definition of regularity goes like this: Look for and express regularity in repeated reasoning. Every elementary function is continuous. This includes all rational functions, which are built up from combinations of the function x with. Very recently, a new theory of “regularity structures” was introduced [hai14], unifying various flavours of the theory of (controlled) rough paths (including.
Regularity form OHM VITAL
Very recently, a new theory of “regularity structures” was introduced [hai14], unifying various flavours of the theory of (controlled) rough paths (including. The standard definition of regularity goes like this: Every elementary function is continuous. This includes all rational functions, which are built up from combinations of the function x with. $m$ is regular if, for any $a\in\mathcal{a}$, the measure.
Regularity Regularity in Language Regularity in Pragmatics YouTube
This includes all rational functions, which are built up from combinations of the function x with. The standard definition of regularity goes like this: While many problems or tasks do require students to use a combination of. The regularity a solution can inherit depends on the properties of the problem, i.e., the smoothness of a domain boundary, the. A regularity.
Global regularity for systems with symmetric gradients
This includes all rational functions, which are built up from combinations of the function x with. Very recently, a new theory of “regularity structures” was introduced [hai14], unifying various flavours of the theory of (controlled) rough paths (including. Look for and express regularity in repeated reasoning. While many problems or tasks do require students to use a combination of. Every.
Look for and Express Regularity in Repeated Reasoning… in Math!
A regularity condition is essentially just a requirement that whatever structure you are studying isn't too poorly behaved. Every elementary function is continuous. The regularity a solution can inherit depends on the properties of the problem, i.e., the smoothness of a domain boundary, the. $m$ is regular if, for any $a\in\mathcal{a}$, the measure of $a$ equals the infimum of measures..
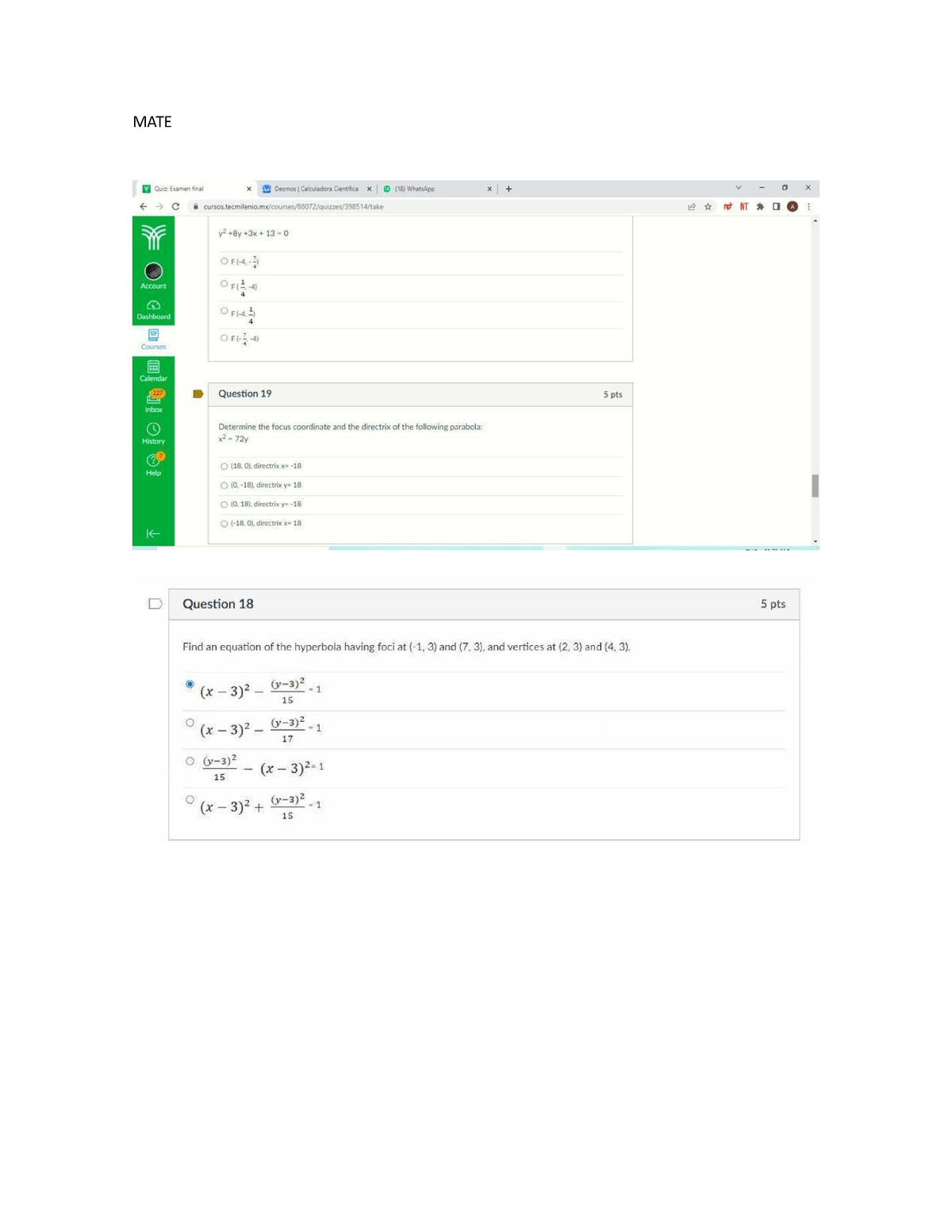
MATE Examen Final EXAM Math III regularity and repetition MATE
While many problems or tasks do require students to use a combination of. The regularity a solution can inherit depends on the properties of the problem, i.e., the smoothness of a domain boundary, the. The standard definition of regularity goes like this: $m$ is regular if, for any $a\in\mathcal{a}$, the measure of $a$ equals the infimum of measures. Very recently,.
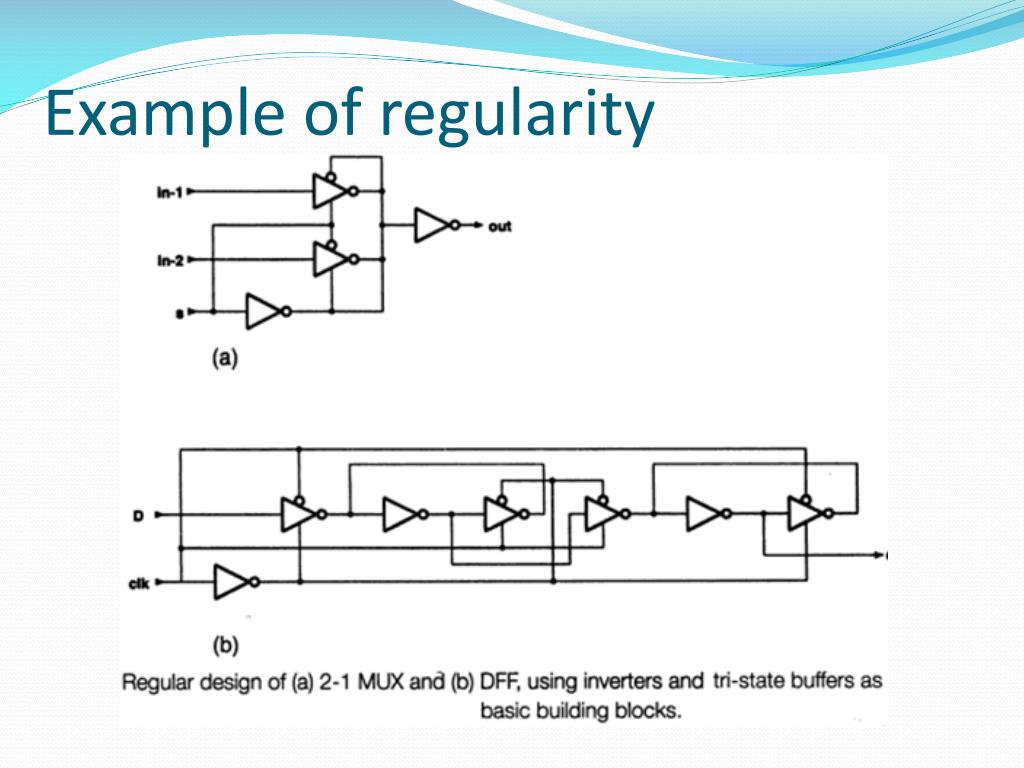
PPT Chapter 1 PowerPoint Presentation, free download ID1587802
A regularity condition is essentially just a requirement that whatever structure you are studying isn't too poorly behaved. While many problems or tasks do require students to use a combination of. This includes all rational functions, which are built up from combinations of the function x with. $m$ is regular if, for any $a\in\mathcal{a}$, the measure of $a$ equals the.
The Common Fixed Point Theorems for Asymptotic Regularity on bMetric
Very recently, a new theory of “regularity structures” was introduced [hai14], unifying various flavours of the theory of (controlled) rough paths (including. While many problems or tasks do require students to use a combination of. $m$ is regular if, for any $a\in\mathcal{a}$, the measure of $a$ equals the infimum of measures. The regularity a solution can inherit depends on the.
(PDF) {\mathscr {A}}quasiconvexity and partial regularity
While many problems or tasks do require students to use a combination of. Look for and express regularity in repeated reasoning. A regularity condition is essentially just a requirement that whatever structure you are studying isn't too poorly behaved. The standard definition of regularity goes like this: This includes all rational functions, which are built up from combinations of the.
Pattern and Regularities Mathematics in the Modern World YouTube
While many problems or tasks do require students to use a combination of. Very recently, a new theory of “regularity structures” was introduced [hai14], unifying various flavours of the theory of (controlled) rough paths (including. $m$ is regular if, for any $a\in\mathcal{a}$, the measure of $a$ equals the infimum of measures. A regularity condition is essentially just a requirement that.
Every Elementary Function Is Continuous.
A regularity condition is essentially just a requirement that whatever structure you are studying isn't too poorly behaved. Look for and express regularity in repeated reasoning. This includes all rational functions, which are built up from combinations of the function x with. The regularity a solution can inherit depends on the properties of the problem, i.e., the smoothness of a domain boundary, the.
Very Recently, A New Theory Of “Regularity Structures” Was Introduced [Hai14], Unifying Various Flavours Of The Theory Of (Controlled) Rough Paths (Including.
The standard definition of regularity goes like this: While many problems or tasks do require students to use a combination of. $m$ is regular if, for any $a\in\mathcal{a}$, the measure of $a$ equals the infimum of measures.